APTITUDE SHORTCUT TRICK for SSC CGL IBPS GATE PSU exam with video.
When people (my students for instance) first see surds they say 'Ugh, we can't do these, THEY LOOK TOO HARD'. This is not uncommon, it is everybody's first impression of surds.
Surds look more difficult than they actually are. In fact, surds are one of the most easiest of sums to do. Once the concept is grasped and the general rules of surds are followed the math problem solving becomes easier to do.
A more mathematical definition of surds is: Surds are irrational numbers. They are numbers which cannot be written exactly.
Here are some general rules to follow regarding surds.
Rules of Surds 1. √a×√b=√ab
2. √a+√b=√a+b (Not True)
3. √b-√a=√b-a (Not True)
4. √a⁄√b=√(a⁄b)
5. √3b=3√b
6. 2√a=√4a
Tip to remember: √4=2
√9=3
√16=4
√25=5
√36=6
Multiplying Surds
Example 1
Work out: √2×√3
Solution: Using the rule above √a×√b=√ab. If we multiply the numbers 2 and 3 to give the answer √6.
Therefore √2×√3=√6
Example 2
Simplify: √12
Solution: using the rule above √a×√b=√ab we can break the number 12 up into smaller numbers such as √4×√3.
(NOTE: There are other ways to do this as 2 and 6 are also factors of 12, the final answer will still be the same)
Using one of the tips above it shows us that √4=2
So simplifying √4×√3 this further, to give us 2×√3
Next to make the answer look more complete and nicer we remove the multiplication sign and put the number 2 next to √3 to give
2√3
So, √12=2√3
Example 3
Work out 2√3×4√5
Solution: Multiply the numbers inside the square root with each other to give 3×5=15 and the outside numbers with each other to give 2×4=8.
Therefore the solution is 2√3×4√5=8√15
Dividing Surds
Example 4
Work out √15÷√5
Solution : Using this rule √a⁄√b=√(a⁄b)
Cancel the numbers down to its lowest form to give
√15⁄√5=√15⁄5=√3
Example 5
Simplify 8√6÷2√3
Solution : Numbers inside the square roots can be divided with each other, as well as the numbers on the outside. So 8÷2=4 and 6÷3=2
Therefore 8√6÷2√3=4√2
Adding Surds
Example 6
Work out √3+√6
Solution : Using the addition rule above √a+√b=√a+b, we can add the numbers 3 and 9 within the square root to give √9
Then, using the tip that √9=3
Therefore √3+√6=√9= 3
Subtracting Surds
Example 7
Work out √7-√2
Solution : Using the subtraction rule above √b-√a=√b-a, we can subtract the 7 and 2 to give 5
Therefore √7-√2=√5
Complex Questions on Surds
Example 8
Solve √-16
Solution can be viewed here on Twitpic, You need a twitter account to view it.
Example 9
Solve 2(x-1)²=-16
Solution can be viewed here on Twitpic, You need a twitter account to view it.
The main thing to remember about surds and working them out is that it is about manipulation. Changing and manipulating the equation so that you get the desired result. Rationalizing the denominator is all about manipulating the algebra expression.
- See more at: http://www.math-problem-solving.com/rules-of-surds.html#sthash.hOkz1jIw.dpuf
When people (my students for instance) first see surds they say 'Ugh, we can't do these, THEY LOOK TOO HARD'. This is not uncommon, it is everybody's first impression of surds.
Surds look more difficult than they actually are. In fact, surds are one of the most easiest of sums to do. Once the concept is grasped and the general rules of surds are followed the math problem solving becomes easier to do.
A more mathematical definition of surds is: Surds are irrational numbers. They are numbers which cannot be written exactly.
Here are some general rules to follow regarding surds.
Rules of Surds 1. √a×√b=√ab
2. √a+√b=√a+b (Not True)
3. √b-√a=√b-a (Not True)
4. √a⁄√b=√(a⁄b)
5. √3b=3√b
6. 2√a=√4a
Tip to remember: √4=2
√9=3
√16=4
√25=5
√36=6
Multiplying Surds
Example 1
Work out: √2×√3
Solution: Using the rule above √a×√b=√ab. If we multiply the numbers 2 and 3 to give the answer √6.
Therefore √2×√3=√6
Example 2
Simplify: √12
Solution: using the rule above √a×√b=√ab we can break the number 12 up into smaller numbers such as √4×√3.
(NOTE: There are other ways to do this as 2 and 6 are also factors of 12, the final answer will still be the same)
Using one of the tips above it shows us that √4=2
So simplifying √4×√3 this further, to give us 2×√3
Next to make the answer look more complete and nicer we remove the multiplication sign and put the number 2 next to √3 to give
2√3
So, √12=2√3
Example 3
Work out 2√3×4√5
Solution: Multiply the numbers inside the square root with each other to give 3×5=15 and the outside numbers with each other to give 2×4=8.
Therefore the solution is 2√3×4√5=8√15
Dividing Surds
Example 4
Work out √15÷√5
Solution : Using this rule √a⁄√b=√(a⁄b)
Cancel the numbers down to its lowest form to give
√15⁄√5=√15⁄5=√3
Example 5
Simplify 8√6÷2√3
Solution : Numbers inside the square roots can be divided with each other, as well as the numbers on the outside. So 8÷2=4 and 6÷3=2
Therefore 8√6÷2√3=4√2
Adding Surds
Example 6
Work out √3+√6
Solution : Using the addition rule above √a+√b=√a+b, we can add the numbers 3 and 9 within the square root to give √9
Then, using the tip that √9=3
Therefore √3+√6=√9= 3
Subtracting Surds
Example 7
Work out √7-√2
Solution : Using the subtraction rule above √b-√a=√b-a, we can subtract the 7 and 2 to give 5
Therefore √7-√2=√5
Complex Questions on Surds
Example 8
Solve √-16
Solution can be viewed here on Twitpic, You need a twitter account to view it.
Example 9
Solve 2(x-1)²=-16
Solution can be viewed here on Twitpic, You need a twitter account to view it.
The main thing to remember about surds and working them out is that it is about manipulation. Changing and manipulating the equation so that you get the desired result. Rationalizing the denominator is all about manipulating the algebra expression.
- See more at: http://www.math-problem-solving.com/rules-of-surds.html#sthash.hOkz1jIw.dpuf
When people (my students for instance) first see surds they say 'Ugh, we can't do these, THEY LOOK TOO HARD'. This is not uncommon, it is everybody's first impression of surds.
Surds look more difficult than they actually are. In fact, surds are one of the most easiest of sums to do. Once the concept is grasped and the general rules of surds are followed the math problem solving becomes easier to do.
A more mathematical definition of surds is: Surds are irrational numbers. They are numbers which cannot be written exactly.
Here are some general rules to follow regarding surds.
Rules of Surds 1. √a×√b=√ab
2. √a+√b=√a+b (Not True)
3. √b-√a=√b-a (Not True)
4. √a⁄√b=√(a⁄b)
5. √3b=3√b
6. 2√a=√4a
Tip to remember: √4=2
√9=3
√16=4
√25=5
√36=6
Multiplying Surds
Example 1
Work out: √2×√3
Solution: Using the rule above √a×√b=√ab. If we multiply the numbers 2 and 3 to give the answer √6.
Therefore √2×√3=√6
Example 2
Simplify: √12
Solution: using the rule above √a×√b=√ab we can break the number 12 up into smaller numbers such as √4×√3.
(NOTE: There are other ways to do this as 2 and 6 are also factors of 12, the final answer will still be the same)
Using one of the tips above it shows us that √4=2
So simplifying √4×√3 this further, to give us 2×√3
Next to make the answer look more complete and nicer we remove the multiplication sign and put the number 2 next to √3 to give
2√3
So, √12=2√3
Example 3
Work out 2√3×4√5
Solution: Multiply the numbers inside the square root with each other to give 3×5=15 and the outside numbers with each other to give 2×4=8.
Therefore the solution is 2√3×4√5=8√15
Dividing Surds
Example 4
Work out √15÷√5
Solution : Using this rule √a⁄√b=√(a⁄b)
Cancel the numbers down to its lowest form to give
√15⁄√5=√15⁄5=√3
Example 5
Simplify 8√6÷2√3
Solution : Numbers inside the square roots can be divided with each other, as well as the numbers on the outside. So 8÷2=4 and 6÷3=2
Therefore 8√6÷2√3=4√2
Adding Surds
Example 6
Work out √3+√6
Solution : Using the addition rule above √a+√b=√a+b, we can add the numbers 3 and 9 within the square root to give √9
Then, using the tip that √9=3
Therefore √3+√6=√9= 3
Subtracting Surds
Example 7
Work out √7-√2
Solution : Using the subtraction rule above √b-√a=√b-a, we can subtract the 7 and 2 to give 5
Therefore √7-√2=√5
Complex Questions on Surds
Example 8
Solve √-16
Solution can be viewed here on Twitpic, You need a twitter account to view it.
Example 9
Solve 2(x-1)²=-16
Solution can be viewed here on Twitpic, You need a twitter account to view it.
The main thing to remember about surds and working them out is that it is about manipulation. Changing and manipulating the equation so that you get the desired result. Rationalizing the denominator is all about manipulating the algebra expression.
- See more at: http://www.math-problem-solving.com/rules-of-surds.html#sthash.hOkz1jIw.dpuf
SURDS AND INDICES
About the topic surds and indices
Surds and Indices play a major role
quantitative aptitude test. There is no competitive exam without the
questions from this topic. We have already learned this topic in our
lower
classes.Even though we have been already taught this topic in our lower
classes, we need to learn some more short cuts which are being used to
solve the problems in the above topic.
The only thing we have to do is,
we need to apply the appropriate short cut and solve the problems in a
limited time. This limited time will be one minute or less than one minute in most of
the competitive exams.
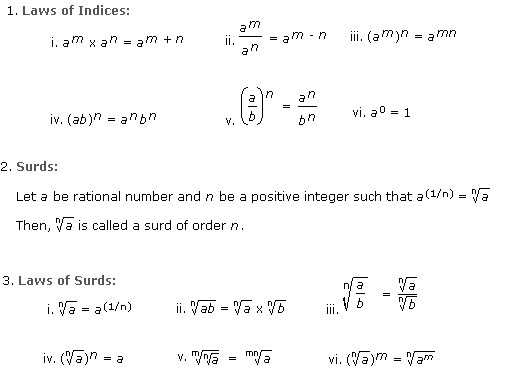
To do problems on surd and indices, we have to remembers the following laws on surds and indices.

Why do students have to study this topic?
Students who are preparing to improve their aptitude skills
and those who are preparing for this type of competitive test must prepare this
topic in order to have better score. Because, today there is no competitive
exam without questions from this topic. Whether a person
is going to write placement exam to get placed or a students is going to write
a competitive exam in order to get admission in university, they must be prepared
to solve problems on indices and surds. This is the reason for why people must study
this topic.
Benefit of studying this topic
As
we mentioned in the above paragraph, a person who wants to get placed in a
company and a students who wants to get admission in university for higher
studies must write competitive exams like placement test and entrance exam. To
meet the above requirement, it is very important to score more marks in the
above mentioned competitive exams. To score more marks, they have to prepare
the topics like this . Preparing this topic would definitely improve their marks in the
above exams. Preparing this topic is not difficult task. We are just going to
remember the stuff that we have already learned in our lower classes
How can students do problems on indices and surds?
Students have to
learn few basic operations in this topic and some additional tricks. Already we
are much clear with the four basic operations which we often use in math. They
are addition, subtraction, multiplication and division. Even though we are much
clear with these four basic operations, we have to be knowing some more stuff
to do the problems which are being asked from this topic in competitive exams.
The stuff which I have mentioned above is nothing but the tricks and shortcuts
which need to solve the problems in a very short time.
Rules of Surds
Rules of surds and examples can be found on this page.When people (my students for instance) first see surds they say 'Ugh, we can't do these, THEY LOOK TOO HARD'. This is not uncommon, it is everybody's first impression of surds.
Surds look more difficult than they actually are. In fact, surds are one of the most easiest of sums to do. Once the concept is grasped and the general rules of surds are followed the math problem solving becomes easier to do.
A more mathematical definition of surds is: Surds are irrational numbers. They are numbers which cannot be written exactly.
Here are some general rules to follow regarding surds.
2. √a+√b=√a+b (Not True)
3. √b-√a=√b-a (Not True)
4. √a⁄√b=√(a⁄b)
5. √3b=3√b
6. 2√a=√4a
√9=3
√16=4
√25=5
√36=6
Multiplying Surds
Example 1
Work out: √2×√3
Solution: Using the rule above √a×√b=√ab. If we multiply the numbers 2 and 3 to give the answer √6.
Therefore √2×√3=√6
Example 2
Simplify: √12
Solution: using the rule above √a×√b=√ab we can break the number 12 up into smaller numbers such as √4×√3.
(NOTE: There are other ways to do this as 2 and 6 are also factors of 12, the final answer will still be the same)
Using one of the tips above it shows us that √4=2
So simplifying √4×√3 this further, to give us 2×√3
Next to make the answer look more complete and nicer we remove the multiplication sign and put the number 2 next to √3 to give
2√3
So, √12=2√3
Example 3
Work out 2√3×4√5
Solution: Multiply the numbers inside the square root with each other to give 3×5=15 and the outside numbers with each other to give 2×4=8.
Therefore the solution is 2√3×4√5=8√15
Dividing Surds
Example 4
Work out √15÷√5
Solution : Using this rule √a⁄√b=√(a⁄b)
Cancel the numbers down to its lowest form to give
√15⁄√5=√15⁄5=√3
Example 5
Simplify 8√6÷2√3
Solution : Numbers inside the square roots can be divided with each other, as well as the numbers on the outside. So 8÷2=4 and 6÷3=2
Therefore 8√6÷2√3=4√2
Adding Surds
Example 6
Work out √3+√6
Solution : Using the addition rule above √a+√b=√a+b, we can add the numbers 3 and 9 within the square root to give √9
Then, using the tip that √9=3
Therefore √3+√6=√9= 3
Subtracting Surds
Example 7
Work out √7-√2
Solution : Using the subtraction rule above √b-√a=√b-a, we can subtract the 7 and 2 to give 5
Therefore √7-√2=√5
Complex Questions on Surds
Example 8
Solve √-16
Solution can be viewed here on Twitpic, You need a twitter account to view it.
Example 9
Solve 2(x-1)²=-16
Solution can be viewed here on Twitpic, You need a twitter account to view it.
The main thing to remember about surds and working them out is that it is about manipulation. Changing and manipulating the equation so that you get the desired result. Rationalizing the denominator is all about manipulating the algebra expression.
- See more at: http://www.math-problem-solving.com/rules-of-surds.html#sthash.hOkz1jIw.dpuf
Rules of Surds
Rules of surds and examples can be found on this page.When people (my students for instance) first see surds they say 'Ugh, we can't do these, THEY LOOK TOO HARD'. This is not uncommon, it is everybody's first impression of surds.
Surds look more difficult than they actually are. In fact, surds are one of the most easiest of sums to do. Once the concept is grasped and the general rules of surds are followed the math problem solving becomes easier to do.
A more mathematical definition of surds is: Surds are irrational numbers. They are numbers which cannot be written exactly.
Here are some general rules to follow regarding surds.
2. √a+√b=√a+b (Not True)
3. √b-√a=√b-a (Not True)
4. √a⁄√b=√(a⁄b)
5. √3b=3√b
6. 2√a=√4a
√9=3
√16=4
√25=5
√36=6
Multiplying Surds
Example 1
Work out: √2×√3
Solution: Using the rule above √a×√b=√ab. If we multiply the numbers 2 and 3 to give the answer √6.
Therefore √2×√3=√6
Example 2
Simplify: √12
Solution: using the rule above √a×√b=√ab we can break the number 12 up into smaller numbers such as √4×√3.
(NOTE: There are other ways to do this as 2 and 6 are also factors of 12, the final answer will still be the same)
Using one of the tips above it shows us that √4=2
So simplifying √4×√3 this further, to give us 2×√3
Next to make the answer look more complete and nicer we remove the multiplication sign and put the number 2 next to √3 to give
2√3
So, √12=2√3
Example 3
Work out 2√3×4√5
Solution: Multiply the numbers inside the square root with each other to give 3×5=15 and the outside numbers with each other to give 2×4=8.
Therefore the solution is 2√3×4√5=8√15
Dividing Surds
Example 4
Work out √15÷√5
Solution : Using this rule √a⁄√b=√(a⁄b)
Cancel the numbers down to its lowest form to give
√15⁄√5=√15⁄5=√3
Example 5
Simplify 8√6÷2√3
Solution : Numbers inside the square roots can be divided with each other, as well as the numbers on the outside. So 8÷2=4 and 6÷3=2
Therefore 8√6÷2√3=4√2
Adding Surds
Example 6
Work out √3+√6
Solution : Using the addition rule above √a+√b=√a+b, we can add the numbers 3 and 9 within the square root to give √9
Then, using the tip that √9=3
Therefore √3+√6=√9= 3
Subtracting Surds
Example 7
Work out √7-√2
Solution : Using the subtraction rule above √b-√a=√b-a, we can subtract the 7 and 2 to give 5
Therefore √7-√2=√5
Complex Questions on Surds
Example 8
Solve √-16
Solution can be viewed here on Twitpic, You need a twitter account to view it.
Example 9
Solve 2(x-1)²=-16
Solution can be viewed here on Twitpic, You need a twitter account to view it.
The main thing to remember about surds and working them out is that it is about manipulation. Changing and manipulating the equation so that you get the desired result. Rationalizing the denominator is all about manipulating the algebra expression.
- See more at: http://www.math-problem-solving.com/rules-of-surds.html#sthash.hOkz1jIw.dpuf
Rules of Surds
Rules of surds and examples can be found on this page.When people (my students for instance) first see surds they say 'Ugh, we can't do these, THEY LOOK TOO HARD'. This is not uncommon, it is everybody's first impression of surds.
Surds look more difficult than they actually are. In fact, surds are one of the most easiest of sums to do. Once the concept is grasped and the general rules of surds are followed the math problem solving becomes easier to do.
A more mathematical definition of surds is: Surds are irrational numbers. They are numbers which cannot be written exactly.
Here are some general rules to follow regarding surds.
2. √a+√b=√a+b (Not True)
3. √b-√a=√b-a (Not True)
4. √a⁄√b=√(a⁄b)
5. √3b=3√b
6. 2√a=√4a
√9=3
√16=4
√25=5
√36=6
Multiplying Surds
Example 1
Work out: √2×√3
Solution: Using the rule above √a×√b=√ab. If we multiply the numbers 2 and 3 to give the answer √6.
Therefore √2×√3=√6
Example 2
Simplify: √12
Solution: using the rule above √a×√b=√ab we can break the number 12 up into smaller numbers such as √4×√3.
(NOTE: There are other ways to do this as 2 and 6 are also factors of 12, the final answer will still be the same)
Using one of the tips above it shows us that √4=2
So simplifying √4×√3 this further, to give us 2×√3
Next to make the answer look more complete and nicer we remove the multiplication sign and put the number 2 next to √3 to give
2√3
So, √12=2√3
Example 3
Work out 2√3×4√5
Solution: Multiply the numbers inside the square root with each other to give 3×5=15 and the outside numbers with each other to give 2×4=8.
Therefore the solution is 2√3×4√5=8√15
Dividing Surds
Example 4
Work out √15÷√5
Solution : Using this rule √a⁄√b=√(a⁄b)
Cancel the numbers down to its lowest form to give
√15⁄√5=√15⁄5=√3
Example 5
Simplify 8√6÷2√3
Solution : Numbers inside the square roots can be divided with each other, as well as the numbers on the outside. So 8÷2=4 and 6÷3=2
Therefore 8√6÷2√3=4√2
Adding Surds
Example 6
Work out √3+√6
Solution : Using the addition rule above √a+√b=√a+b, we can add the numbers 3 and 9 within the square root to give √9
Then, using the tip that √9=3
Therefore √3+√6=√9= 3
Subtracting Surds
Example 7
Work out √7-√2
Solution : Using the subtraction rule above √b-√a=√b-a, we can subtract the 7 and 2 to give 5
Therefore √7-√2=√5
Complex Questions on Surds
Example 8
Solve √-16
Solution can be viewed here on Twitpic, You need a twitter account to view it.
Example 9
Solve 2(x-1)²=-16
Solution can be viewed here on Twitpic, You need a twitter account to view it.
The main thing to remember about surds and working them out is that it is about manipulation. Changing and manipulating the equation so that you get the desired result. Rationalizing the denominator is all about manipulating the algebra expression.
- See more at: http://www.math-problem-solving.com/rules-of-surds.html#sthash.hOkz1jIw.dpuf




0 comments:
Post a Comment